Unveiling the Elegance of Fluid Dynamics: Mastering Bernoulli’s Equation Derivation for a Deeper Dive into the Art of Fluid Mechanics
Introduction
Fluid mechanics is a branch of physics that deals with the study of fluids’ behaviour under various conditions. Fluid flow, a fundamental concept within fluid mechanics, plays a crucial role in understanding how liquids and gases move and interact. In this article, we will delve into the definition of fluid flow, explore different types of fluid flow, discuss when fluid flow becomes laminar, and finally, derive Bernoulli’s equation – a fundamental principle in fluid dynamics.
Fluid Flow Definition
Fluid flow refers to the movement of liquids and gases through various mediums. It is a phenomenon that can be observed in a variety of contexts, from the movement of water through pipes to the flow of air around airplane wings. Fluid flow is governed by a set of principles and equations, one of which is Bernoulli’s equation.
Types of Fluid Flow
Fluid flow can be classified into two main types: laminar flow and turbulent flow. Laminar flow occurs when a fluid moves in smooth layers, without significant mixing between adjacent layers. This type of flow is characterised by its regular and predictable behaviour. On the other hand, turbulent flow is characterised by chaotic and irregular motion, in which mixing and eddies exist within the fluid.
The Flow of Fluid Will Be Laminar When?
If the Reynolds number (Re) is low, the flow of the fluid will be laminar. The Reynolds number is a dimensionless quantity that determines whether a flow is laminar or turbulent. It is calculated using the density, velocity, viscosity and characteristic length of the fluid.
Classification of Fluid Flow
Fluid flow can also be classified based on factors such as the direction of flow, the viscosity of the fluid, and the presence of external forces. Steady flow involves a consistent flow rate and velocity, while unsteady flow exhibits fluctuations over time. Incompressible flow refers to fluid flow where the density remains constant, and compressible flow involves a variation in density.
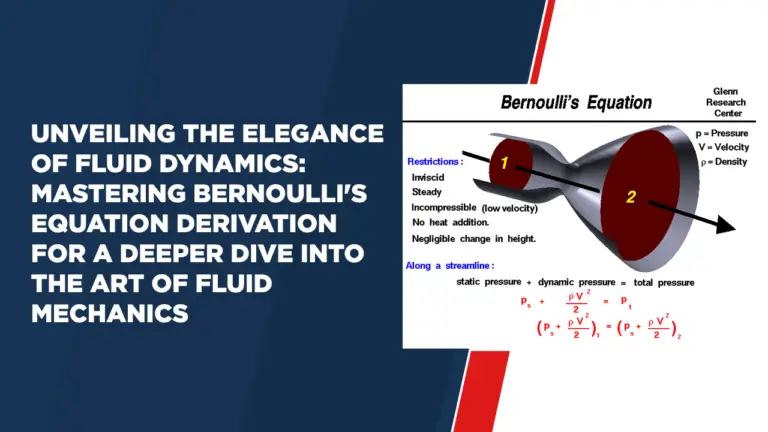
Derivation of Bernoulli’s Equation
Bernoulli’s equation is a fundamental principle in fluid mechanics that describes the relationship between pressure, velocity, and height in a fluid flow. It is derived from the principle of conservation of energy along a streamline. The equation can be expressed as:
P+ρv2/2+ρgh=constant
where P is the pressure, ρ is the density, v is the velocity, g is the acceleration due to gravity, and h is the elevation.
Conclusion
Understanding fluid flow and its underlying principles is essential in a variety of fields, from engineering to meteorology. By understanding the classification of fluid flow, recognizing laminar and turbulent behaviours, and deriving Bernoulli’s equation, we gain insight into the complex behaviour of fluids in motion. Fluid mechanics remains a fascinating field of study with practical applications that affect our daily lives.